Understanding Y As A Function Of X: A Comprehensive Guide

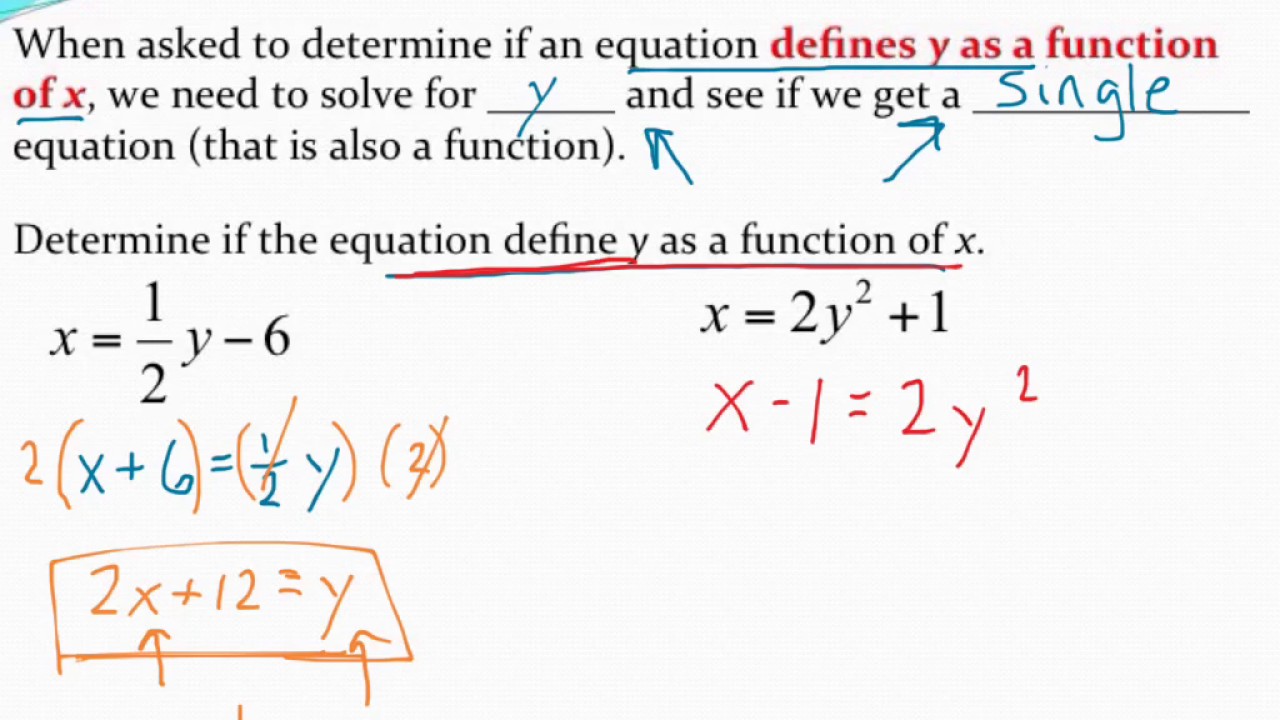
In the realm of mathematics, the concept of "y as a function of x" is fundamental. It serves as the backbone for various disciplines including algebra, calculus, and even statistics. Understanding how one variable (y) can be expressed in terms of another (x) opens the door to solving complex problems and modeling real-world scenarios. In this article, we will delve deep into this concept, exploring its significance, applications, and the mathematical principles that govern it.

Whether you are a student struggling with your math homework or a professional looking to brush up on your skills, grasping the relationship between y and x is crucial. This guide will not only provide you with the theoretical knowledge but also practical examples and applications that highlight how this function is utilized in everyday life.

Throughout this article, we will define key terms, explore different types of functions, and provide visual aids to enhance your understanding. By the end of this comprehensive guide, you will have a clearer grasp of y as a function of x, along with its implications in various fields.

Table of Contents

• 1. Definition of a Function
• 2. Types of Functions
• 3. Graphing Functions: Y as a Function of X
• 4. Practical Examples
• 5. Applications in Real Life
• 6. Common Mistakes in Understanding Functions
• 7. Importance of Understanding Y as a Function of X
• 8. Conclusion

1. Definition of a Function

A function is a relation that uniquely associates members of one set with members of another set. In simpler terms, a function describes how one quantity depends on another. When we say "y as a function of x," we mean that for every input value of x, there is a corresponding output value of y. This relationship can be expressed in various forms, including equations, graphs, or tables.

1.1 Mathematical Notation

The notation f(x) is commonly used to denote a function where 'f' represents the function and 'x' is the independent variable. The output, or dependent variable, is denoted as f(x) or y. Thus, we can express the relationship as:

y = f(x)

2. Types of Functions

Functions can be categorized into different types based on their characteristics. Understanding these types is essential for grasping the concept of y as a function of x.

2.1 Linear Functions

A linear function is a function that produces a straight line when graphed. It has the general form:

y = mx + b

where 'm' is the slope and 'b' is the y-intercept.

2.2 Quadratic Functions

A quadratic function is a second-degree polynomial function, represented as:

y = ax² + bx + c

This function produces a parabolic graph.

2.3 Exponential Functions

An exponential function is characterized by its rapid growth rate and can be written as:

y = ab^x

where 'a' is a constant and 'b' is the base of the exponential.

2.4 Trigonometric Functions

Trigonometric functions, such as sine and cosine, relate angles to side ratios in a right triangle. They are periodic functions and can also be expressed as:

y = sin(x) or y = cos(x)

3. Graphing Functions: Y as a Function of X

Graphing is a powerful tool to visualize the relationship between y and x. Different functions exhibit unique shapes, which can provide insights into their behavior and characteristics.

3.1 Understanding the Cartesian Plane

The Cartesian plane consists of two perpendicular axes: the x-axis (horizontal) and the y-axis (vertical). Each point on the plane is identified by its coordinates (x, y).

3.2 Plotting Functions

To graph a function, we can follow these steps:

• Choose values for the independent variable (x).
• Calculate the corresponding values of the dependent variable (y).
• Plot the points on the Cartesian plane.
• Connect the points to visualize the function.

4. Practical Examples

Let's explore some practical examples to illustrate y as a function of x in various contexts.

4.1 Example 1: Linear Function

If we have the equation:

y = 2x + 3

For x = 1, y = 2(1) + 3 = 5. For x = 2, y = 2(2) + 3 = 7. Plotting these points shows a straight line.

4.2 Example 2: Quadratic Function

Consider the quadratic function:

y = x² - 4

This function will produce a parabolic graph, with its vertex at the point (0, -4).

5. Applications in Real Life

The concept of y as a function of x is not just limited to mathematical theories; it has practical applications in various fields.

5.1 Physics

In physics, velocity can be expressed as a function of time. For example, if a car accelerates, its distance traveled can be modeled as:

d(t) = vt + (1/2)at²

where d is distance, v is initial velocity, a is acceleration, and t is time.

5.2 Economics

In economics, supply and demand can be represented as functions. The price (y) can be expressed as a function of quantity supplied (x):

p = f(q)

6. Common Mistakes in Understanding Functions

It's essential to be aware of common pitfalls when working with functions.

6.1 Confusing Independent and Dependent Variables

Many students confuse independent and dependent variables. Remember, x is the input (independent), and y is the output (dependent).

6.2 Overlooking Domain and Range

Every function has a domain (possible x values) and a range (possible y values). Failing to recognize these can lead to incorrect interpretations.

7. Importance of Understanding Y as a Function of X

Grasping the concept of y as a function of x is vital for various reasons:

• It enhances problem-solving skills.
• It provides a foundation for advanced mathematics.
• It enables better understanding of scientific and economic principles.

8. Conclusion

In conclusion, understanding y as a function of x is a critical mathematical concept that permeates various disciplines. From its definition to practical applications, we have explored the multifaceted nature of this relationship. We encourage you to apply this knowledge in real-world scenarios and continue your mathematical journey.

If you found this article helpful, please leave a comment, share it with others, or explore more articles on our site.

Thank you for reading, and we look forward to your next visit!